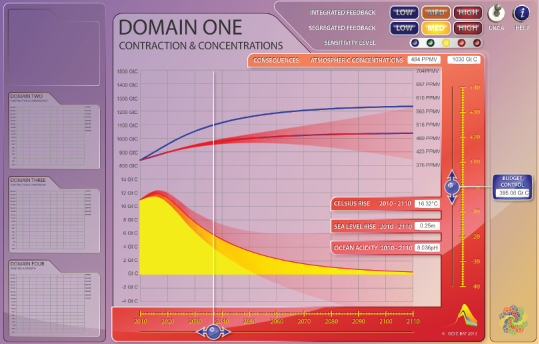
BACK TO CBAT Graphic User Interface [GUI]

BACK TO ALL INFO POINTS DOMAIN 1

The Equation That Couldn't Be Solved
How Mathematical Genius Discovered the Language of Symmetry
By Mario Livio
The book is partly a eulogy for Evariste Galois.
But this beautiful section [quoted from pages 204 205] draws attention to one of the exceptional down-stream insight-consequences of Galois' 'Group Theory'.
"Oddly enough, in spite of his incredible intuition and deep insights in physics, Einstein's attitude toward pure mathematics was at first rather lukewarm. As a student in Zurich, his less-than-perfect attendance in the math classes of mathematician Hermann Minkowski (I864-1909) gained him the title ‘lazy dog.‘ Through an ironic twist of history, once Einstein published his theory of special relativity, it was none other than Minkowski himself who used symmetry to put the theory on a firm mathematical basis. Minkowski showed that space and time may be ‘rotated’ as a four-dimensional entity, just as a sphere can be rotated in three-dimensional space. More important, in the same way that a sphere is symmetric (i.e., it does not change) under rotation through any angle about any axis, Einstein's special relativity equations are symmetric (‘covariant‘ in the physics lingo) under these space-time rotations. This remarkable symmetry of the equations has become known as Lorentz covariance, after the Dutch physicist Hendrik Antoon Lorentz (I853-1928), who first described these transformations in 1904.
You will probably not be too surprised to hear that the collection of all the symmetry transformations of the Minkowski space-time forms a group, similar to the group of ordinary rotations and translations in three dimensions. This group is known as the Poincare group, after the outstanding French mathematician who refined the mathematical basis of special relativity. Suspicious at first (‘ever since the mathematicians have invaded the relativity theory, I myself no longer understand it‘), Einstein slowly began to grasp the incredible power of symmetry.
lf the laws of nature are to remain unchanged for moving observers, not only do the equations describing these laws need to obey Lorentz covariance, the laws themselves may actually be deduced from the requirements of symmetry.
This profound realization has literally reversed the logical process that Einstein (and many of the physicists who followed him) employed to formulate the laws of nature. Instead of starting with a huge collection of experimental and observational facts about nature, formulating a theory, and then checking whether the theory obeys some symmetry principles, Einstein realized that the symmetry requirements may come first and dictate the laws nature has to obey."
Perfect . . . .
CBAT - FULL 4 DOMAIN MOCK-UP
Some Responses to CBAT so far

Some Support for the Classic C&C Concept over the years.