'Stringularity' and the musical derivation of 'Phi'- & WHAT ABOUT CLIMATE CHANGE> >.

 

Click logo to return to 'links-page'

John Archibald Wheeler said, ”It is my opinion that everything must be based on a simple idea and
it is my opinion that this idea, once we have finally discovered it, will be so compelling, so beautiful,
that we will say to one another, yes, how could it have been any different.”

Pythagoras string experiment shows the basis of why we can say: -


  • The rate of vibration heard covers three 'octaves' in super-position, through 100 Hz, 200 Hz, 300 Hz, 400 Hz, 500 Hz, 600 Hz, 800 Hz, revealing pure harmony.
  • The rate of vibration seen is slowed down by a factor of 100 so it can actually be seen in detail rather than as a 'blur' at real-time speed (Hz measure space-time).

This phenomenon is neither 'top-down' nor 'bottom-up'. It is true at all scales of all dimensions. It leads directly to well-tempering and the 'Well Tempered Climate Accord' (WTCA). Music - the universe itself - is impossible without this. Equally UNFCCC-Compliance is impossible without WTCA. "This is not just the 'right way, it is the only way" (GLOBE Point 16)

"All life aspires to the condition of music."

The calculating spreadsheet for this model is down-loadable here


The value of Phi emerges as a universal-constant at exactly the Phi-Point between steps 12 &13 of the Pythagorean Comma
regardless of the base number entered by the user.



************************************************************************

The ‘Stringularity’ Hypothesis

How Pythagoras' simple string experiment, conducted in Crotone around 530 BC,
embeds a per-unit-time-based-derivation of the beautiful and irrational 'Phi'.

************************************************************************

The value of ‘Phi’ (.618…) is irrational. It is commonly derived from a ‘quadratic equation’
((the square root of 5 + 1)/2) or the Fibonacci Series (as in the Pentagon:Pentangle).

However, the value of ‘Phi’ can be quantized per-unit-time (Hz) from measuring and back-feeding
the differences in the phenomenon known as the ‘Pythagorean Comma’. Derived from ‘Stringularity’,
‘Phi’ is a point on a curve at the ‘Phi-point’, between steps 12 and 13 of the Pythagorean Comma.

It is constant, anywhere from nano-to-macroscale. So, using the derivation of ‘Phi’ as a function of
steps per-unit-time, can be a relevant step in changing the cognitive paradigm where particles in
wave-fields are only probability perceptions still regarded as the ‘quantum measurement problem’.

********************************************************************

The ‘Stringularity’ Hypothesis; the ‘path-integral’ of Pythagoras’ String Experiment

The now famous string experiment of Pythagoras (~ 530 BCE) demonstrates that: -

When we take a string of constant length and constant tension tuned at 100 Hz

And halve the length of the string, the frequency (Hz) doubles from 100 Hz to 200 Hz

And that if we third the length of the string the frequency (Hz) trebles to 300 Hz etc . . . .

As the path-integral basis of the universally constant ‘harmonic series’, these steps start with: -

the ‘Fundamental’

the ‘Perfect Octave’

the ‘Perfect Octave plus the Perfect Fifth’ etc . . .
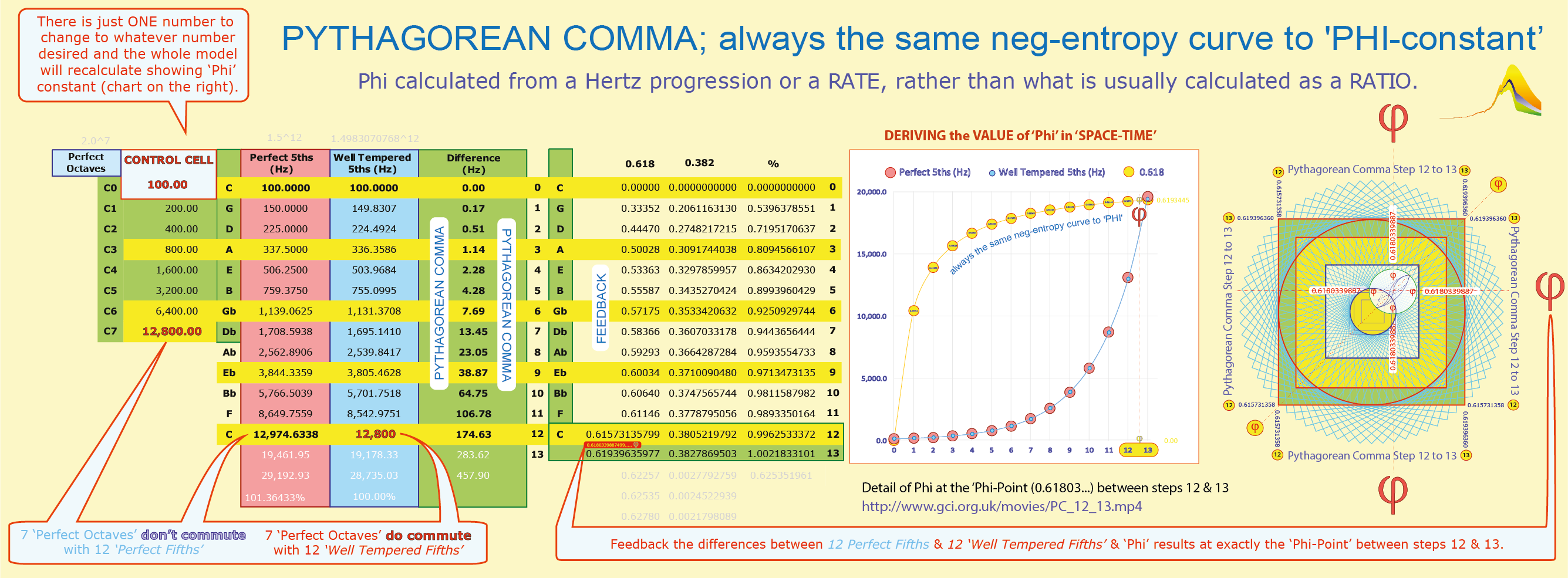
sub-division by any rational number will produce a 'harmonic' in this series . . . .

The Pythagorean Comma (see chart above)

Proceeding from above, the Pythagorean Comma emerges as a growing ‘gap’: -

when we sequence seven ‘Perfect Octaves’ in Hertz (Hz), in the example shown we go through
seven doublings 1 (each enlarging by 200%), from 100 Hz to 12,800.00 Hz,

when we sequence twelve ‘Perfect Fifths’ we go in the example shown we go through 12 steps
(each enlarging by 150%) from 100 Hz to 12,974.63 Hz,

it becomes clear that seven Perfect Octaves (12,800.00 Hz) do not ‘commute’ exactly with
twelve ‘Perfect Fifths’ 12,974.63 Hz. At this ‘twelfth-step’, the gap is 174.63 Hz (1.345...%)

Well-Tempered Tuning (see chart above)

In order to get the twelve Perfect Fifths to commute with the seven Perfect Octaves,
the Perfect Fifths (enlarging at 150%) are ‘Well Tempered’, enlarging at 149.83070768…%)

The result of adopting this procedure is that twelve Well-Tempered Fifths commute exactly
with seven Perfect Octaves (at 12,800 Hz), giving rise to what is called 'Well Tempered Tuning' (3, 4,)

The Hz Differences between Perfect and Well-Tempered Fifths lead to ‘Phi’

When step-by-step, we subtract 12 Well Tempered Fifths from 12 Perfect Fifths,
a sequence of differences between the two paths is the result (see table above),

When step-by-step, we back feed these differences into the sequence, so the largest
difference is divided into the smaller difference that precedes it etc . . . (see table above),

A twelve step sequence with negative curvature (concavity) is revealed, decreasing from
0.615731…. just below the value of ‘Phi’, to 0.000 (see table above),

When we go one step further and start at the thirteenth step of the Pythagorean Comma,
the difference is 283.62 Hz, yielding a value 0.6199396…. just above the value of ‘Phi.

‘Phi’ is exactly at the Phi-Point between per-unit-time steps 12 & 13 of the Pythagorean Comma

The value of ‘Phi’ is on the overall curve at exactly the Phi-point between steps 12 and 13
of the Pythagorean Comma http://www.gci.org.uk/movies/PC_12_13.mp4

Exponential growth curves at 200% 150% and 149.83070768…% etc are positively governed
by acceleration (convexity) and unfold without limit towards infinity.

Path-integral to that, the growth curve to ‘Phi’, is governed by deceleration within the
feedback limits or concavity that define it, countervailing the convexity of acceleration.

Deriving the value of ‘Phi’ from measuring the path-integral per-unit-time/space of
‘Stringularity’ is distinct from the time-free quadratic equation and Fibonacci Series.

This path-integral procedure gives rise to an array of time-based features which suggest corroboration.

The beauty and the deceptive simplicity of ‘Phi’ underlies the structure, the sequencing,
the symmetry and the curvature of natural phenomena, from the nanoscale to the macroscale.

Could this 'musical-derivation' of ‘Phi’ be a simple yet beautiful idea that helps to answer Erwin Schrodinger’s
famous question, ‘What is Life’? Might it underlie a ‘cognitive framework’ that would help better to understand
the wave:particle perceptions-dichotomy, the path-integral complexity, the moment:momentum continuity and
life:death challenges that will always face us?

Concomitant corroboration . . . . ?

Phi squares the circle

Khufu Squares the Circle

The equivalence of Phi and Pi

Phyllotaxis - the spirallic, nested, emergent, folded, ubiquitous, awesome Phi

The 'symmetry binding' properties of Hz-derived Phi integration

the relevance of this to the Standard Model when introducing the Higgs Boson

Phi-Spiral (vortex) 'black-holes' from excitons to galaxies

Footnotes

1. Seven Perfect Octaves (or Hz doublings) is about the length of a modern grand-piano keyboard.
2. This ‘non-commutation’ phenomenon has been recognized for Millennia.
Unaffected by issues of scale and whatever base Hz value is set for ‘doublings,
if 12 Well Tempered Fifths are 100%, 12 Perfect Fifths are always 101.36433…%.

3. In musical narrative, the purpose of adopting ‘Well Tempering’ was to enable music
to be written and played in all the keys (of all the 12 semitone steps in the octave)
so they are all equally ‘in-tune’ and where modulating between these keys these is
smoothed by the equality of the twelve semitone steps in the Perfect Octaves arising. The Well-Tempered Climate Accord is based on precisely this logic.

4. The classical example of this is the Well-Tempered Clavier of JS Bach (1721).